Albert Einstein called it the 8th Wonder of the World (probably because it seems to possess magic powers). It is a phenomenon that can make millionaires out of just about anyone and to top it off, it requires little work on your behalf. It is compound interest.
Compound interest means that each time interest is paid, it is added to (or compounded into) the principal and thereafter also earns interest – accumulation of interest on the interest. Compound interest is one of the most basic and powerful concepts that can enhance the outcome of your investments.
To illustrate compound interest, we will use the following examples:
Consider an example where you invest $100 into a term deposit that pays you 10% at the end of the year. At the end of the year you will receive your $10, plus the invested $100 back, leaving you a total of $110.
- If you do not compound your interest in year two, you will invest your $100 and get another $10 back. So after 2 years you have $120
- If you compound your interest, you will re-invest the $110. After 2 years you'll now have $121. That is 5% higher return than if you did not compound the interest (i.e. $20 return verus $21 return).
There are two main considerations when you think about compound interest:
- Time. The longer you compound, the greater the effect on your investment.
- The more regular your interest repayments are, the greater the effect of compound interest (i.e. annual, semi-annually, quarterly, monthly and continuously). It is to your benefit to select an investment that compounds more regularly. Many of the online savings accounts is compounded daily
Here's another example. The magic of compounding interest (respect to time)
Lets assume Sue saves $200 a month for 5 years (she will hand over $12,000 over the 5 years). With the help of compound interest Sue will have $14,369.15. (derived using a compound interest calculator)
* Assume Sue is receiving 7% interest.
Now this is where it gets interesting. What will happen if Sue saves for 20 years? How much will she have saved? Most people will assume a little under $60,000 (~15k X 4). However after 20 years, Sue will have saved $104,751.14!
Now let’s get a little greedy and assume Sue saves for 50 years. What now? $300,000? At the end of 50 years Sue is worth $1,100,720.27, yes a millionaire!
Here is another example.
Lets look at 3 different investors. Investor A is the Smart Investor, Investor B is the Late Investor and Investor C the Hard Investor.
Investor A: Smart Investor, starts saving $200/month at age 20 for 10 years
Investor B: Late Investor, starts saving $200/month at age 30 for 10 years
Investor C: Hard Investor, starts saving at $200/month at age 30 until retirement (65, 35 years)
*Assume 7% interest
Lets have a look at the table below:
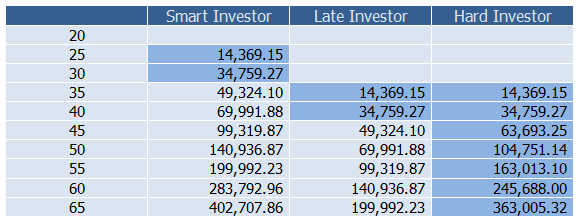
The shaded area indicates the years in which an additional $200/week was added into the savings. The graph shows:
- By investing earlier, by 10 years, Smart Investor has more than doubled Late Investor.
- Hard Investor started saving 10 years behind Smart Investor and even though Hard Investor saved an extra $200/month for 25 years more (35 years compared to 10 years), Hard Investor still has $39,702.54 less than Smart Investor.
The moral of the story? The sooner you start saving the quicker you will see power of compound interest!
As mentioned above there are 2 main considerations when we talk about compound interest, time (which we have just talked about) and frequency of payment. Let’s look at frequency of payment a little closer.
The magic of compounding interest (frequency of payments)
If you invested $100 at a rate of 10% for 3 years. The tables below illustrates the effect of compounding periods :
- The more frequent the interest periods, the greater the effect of compound interest.
- The below tables illustrate the effect of your investment value after a three year period, for your initial investment of $100.
- The first table illustrates interest getting paid annually, at the end of the year. At the end of year 3, you will receive $133.10. The second table illustrates interest being paid twice a year. This means 5% is paid after 6 months (and assuming you reinvest the interest that you have received) another 5% is paid after 12 months, etc. After the 3 year timeframe, you will have $134.01. The final table illustrates your investment paying interest quarterly (after every 3 months). It also illustrates that the greater the frequency of interest repayments, the greater the effect of compounding interest. The investment after 3 years is $1.39 greater than when your interest is paid annually.
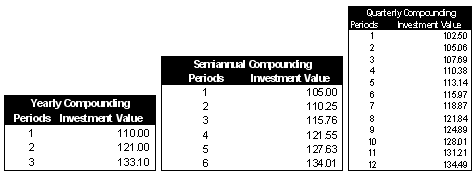
For no larger risk, you are getting $1.39 more after 3 years. The effect of compound interest is substantial for larger investments. Therefore, ensure you check the frequency of interest payments when investing in deposit accounts.